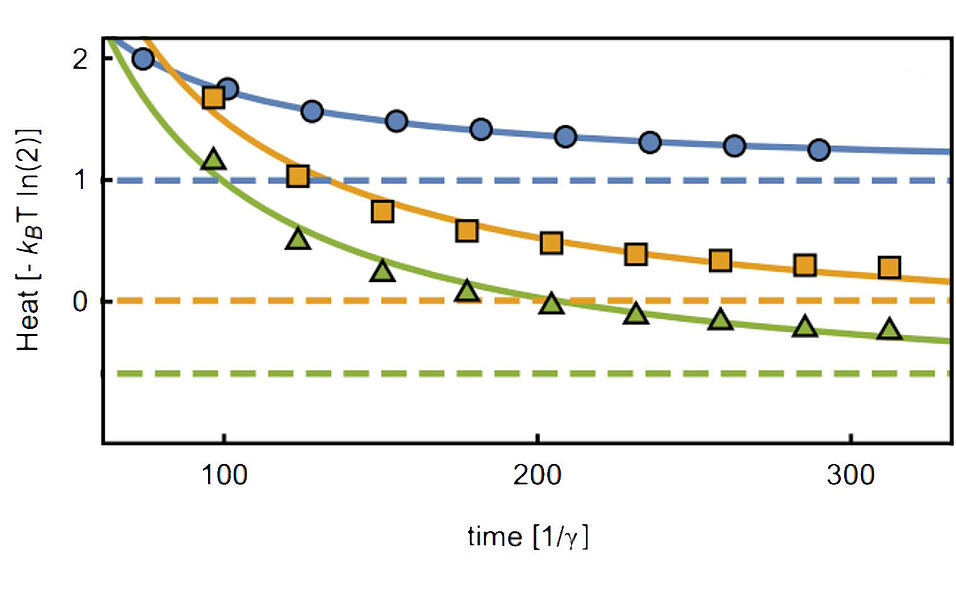
Landauer’s principle states that information erasure requires heat dissipation. While Landauer’s original result focused on equilibrium memories, we here investigate the reset of information stored in a nonequilibrium state of a symmetric two-state memory. We derive a nonequilibrium generalization of the erasure principle and demonstrate that the corresponding bounds on heat and work may be reduced to zero. We further introduce reset protocols that harness energy and entropy of the initial preparation and so allow to reach these nonequilibrium bounds. We finally provide numerical simulations with realistic parameters of an optically levitated nanosphere memory that support these findings. Our results indicate that local dissipation-free information reset is possible away from equilibrium.